
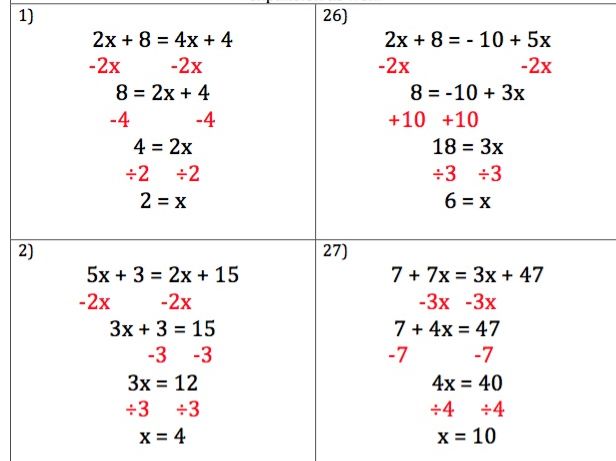
One of the unknown factors (for example x) and Substitution (elimination of variables): It consists in isolating In this section we will resolve linear systems of two equations and two unknown factors with the methods we describe next, which are based on obtaining a first degree equation (a linear equation). To solve consistent dependent a system, we need at least the same number of equations as unknown factors.

We will not speak about other kinds of systems. If there is only one solution (one value for each unknown factor, like in the previous example), the system is said to be a consistent dependent system. There is not always a solution and even there could be an infinite number of solutions. For example,Ĭonsists in finding a value for each unknownįactor in a way that it applies to all the What these equations do is to relate all the unknown factors amongt themselves.
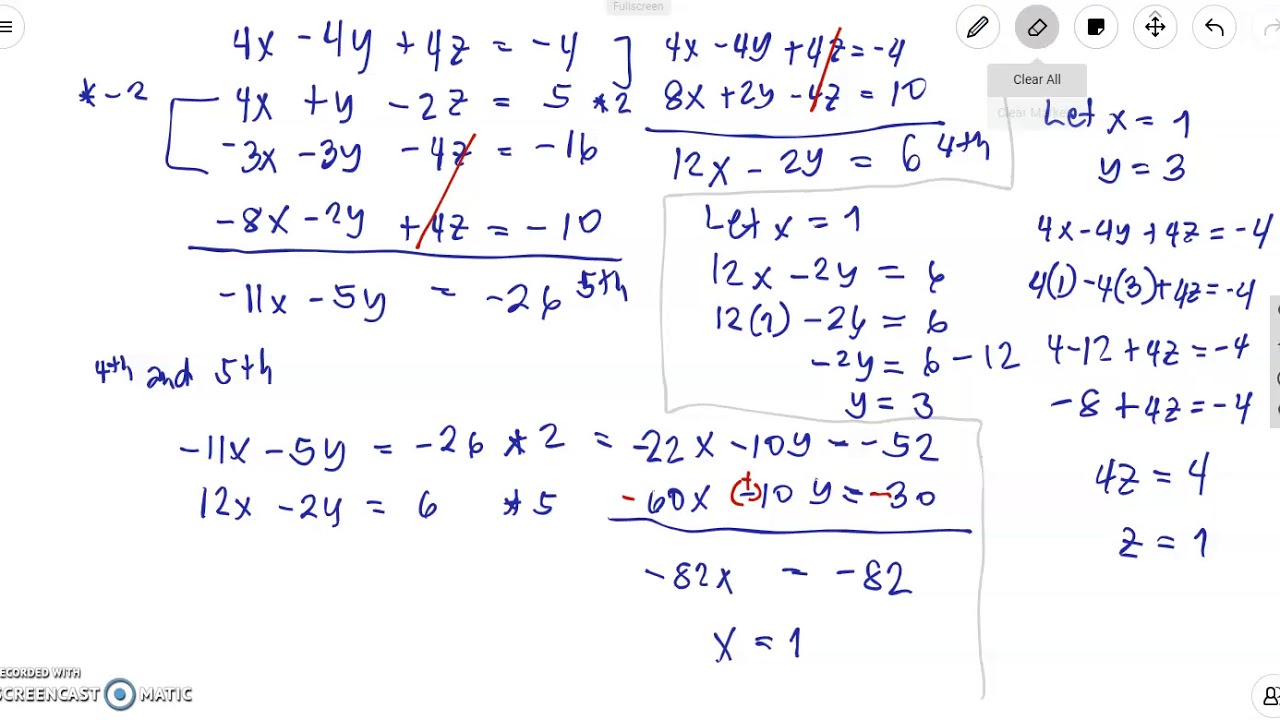
The unknown factors appear in various equations, but do not need to be in all of them. Solving systems of equations is a very general and important idea, and one that is fundamental in many areas of mathematics, engineering and science.4 resolved systems of linear equations by substitution, addition and equalizationĪ system of linear equations (or linear system) is a group of (linear) equations that have more than one unknown factor. Going further, more general systems of constraints are possible, such as ones that involve inequalities or have requirements that certain variables be integers. These possess more complicated solution sets involving one, zero, infinite or any number of solutions, but work similarly to linear systems in that their solutions are the points satisfying all equations involved. More general systems involving nonlinear functions are possible as well. Systems of linear equations involving more than two variables work similarly, having either one solution, no solutions or infinite solutions (the latter in the case that all component equations are equivalent). The system is said to be inconsistent otherwise, having no solutions. If all lines converge to a common point, the system is said to be consistent and has a solution at this point of intersection. In the case of two variables, these systems can be thought of as lines drawn in two-dimensional space. Systems of linear equations are a common and applicable subset of systems of equations. To solve a system is to find all such common solutions or points of intersection. The solutions to systems of equations are the variable mappings such that all component equations are satisfied-in other words, the locations at which all of these equations intersect. What are systems of equations? A system of equations is a set of one or more equations involving a number of variables.
#Steps for 3 equation systems generator#
Get immediate feedback and guidance with step-by-step solutions and Wolfram Problem Generator
#Steps for 3 equation systems how to#
Here are some examples illustrating how to ask about solving systems of equations. To avoid ambiguous queries, make sure to use parentheses where necessary. Additionally, it can solve systems involving inequalities and more general constraints.Įnter your queries using plain English. It can solve systems of linear equations or systems involving nonlinear equations, and it can search specifically for integer solutions or solutions over another domain. Wolfram|Alpha is capable of solving a wide variety of systems of equations. Equation 4: Compute A powerful tool for finding solutions to systems of equations and constraints


 0 kommentar(er)
0 kommentar(er)
